When doing vibration analysis, the signals received from accelerometers in time waveform are examined in different domains by various mathematical transformations. The most common of these is the spectral analysis that we study in the frequency domain. Spectral analysis generates the vibration signature of the machines as well. When we put the raw signal into the Fourier Transform, we get the “Spectrum”. The spectrum provides information about the periodic behavior in the raw signal. We examine the periodicity that occurs with certain frequency spacings in the spectrum with Cepstral Analysis.
The Cepstral Analysis is a tool for detecting periodicity in the frequency spectrum and it is mainly used in pitch detection, radar and sonar applications, speech analysis, and diagnostics. In vibration analysis, it can be used to identify gearbox faults, especially where the carrier frequencies and equally-spaced sidebands in the spectrum are produced. The presence of such sidebands is intriguing in the analysis of gearbox vibration signals. Because a series of errors tend to cause modulation of the vibration pattern that results from tooth meshing, and this modulation leads to sidebands in the frequency spectrum. Sidebands are grouped around gear mesh frequency and harmonics as intervals in multiples of modulating frequencies, and determining these modulation frequencies is very useful in diagnosing the error. At this point, it will be useful to talk about gear faults in terms of making sense of the subject.
Gear Faults
While a gearbox may cause harmonics in the vibration spectrum at normal low drive frequencies, it is also a rotating piece of equipment that exhibits a lot of activity in the high-frequency region because of the gear and bearing impacts. Gear faults are generally predicted by the gear mesh frequency and the presence of its harmonics.
Gear Mesh Frequency (GMF) = Number of Teeth x RPM
The gear mesh frequency has operating speed sidebands according to the shaft speed to which the gear is attached. For this reason, vibration data in the gearbox should be taken separately from each shaft bearing. Gearbox spectrums contain a series of frequencies due to different gear mesh frequencies and their harmonics. The amplitude of all peaks in the spectrum is low and if the gearbox is in good condition, the natural frequency of any gear is not excited. GMF and its harmonics provide information on failure modes as the presence of their surrounding sidebands progresses and their amplitudes increase. At this point, that is, at the point of determining the presence and amplitude of sidebands, the Cepstral Analysis is useful.
Definitions
The cepstrum concept was first introduced in 1963 by BP Bogert, MJ Healy and JW Tukey. It acts as a tool for analyzing periodic structures in frequency spectrums. Such effects are related to noticeable echoes or reflections in the signal or the generation of harmonic frequencies. Mathematically, it deals with the deconvolution problem of signals in the frequency domain.
The terms “quefrency”, “cepstrum”, “saphe”, “gamnitude” were defined by the authors by rearranging the words spectrum, frequency, spectrum, phase, magnitude.
The cepstrum can be obtained in two ways:
-Power Cepstrum
-Complex Cepstrum
In the Power Cepstrum, the logarithm is taken from the power spectrum. In the Complex Cepstrum, the logarithm is taken from the spectrum generated by the Fourier Transform.
Quefrency is the independent variable of the cepstrum and has the dimensions of time as in the case of the autocorrelation. The quefrency in seconds is the reciprocal of the frequency spacing in Hz in the original frequency spectrum of a particular periodically repeating component. Just as the frequency in a normal spectrum says nothing about absolute time but only about repeated time intervals (the periodic time), the quefrency only gives information about frequency spacings, not about absolute frequency.
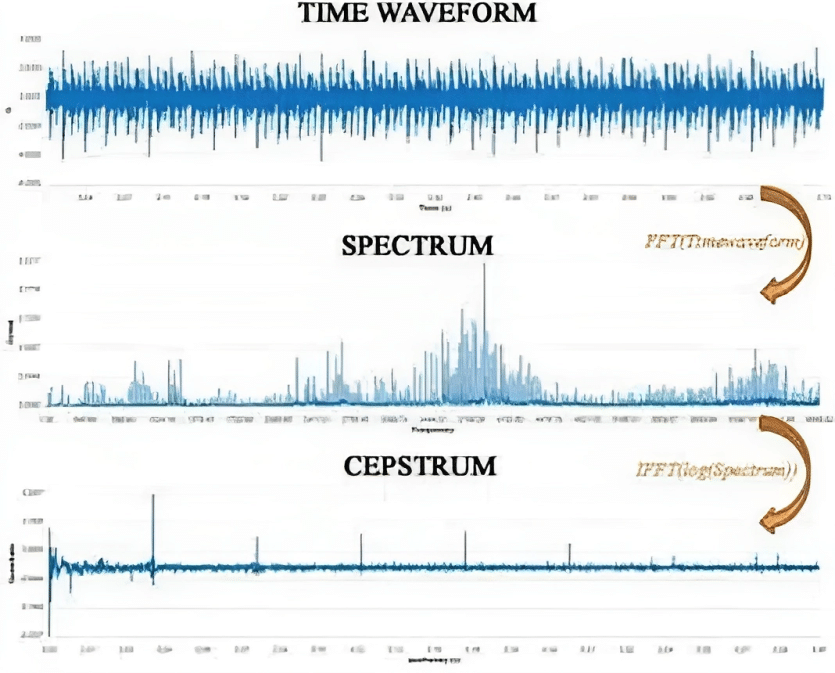
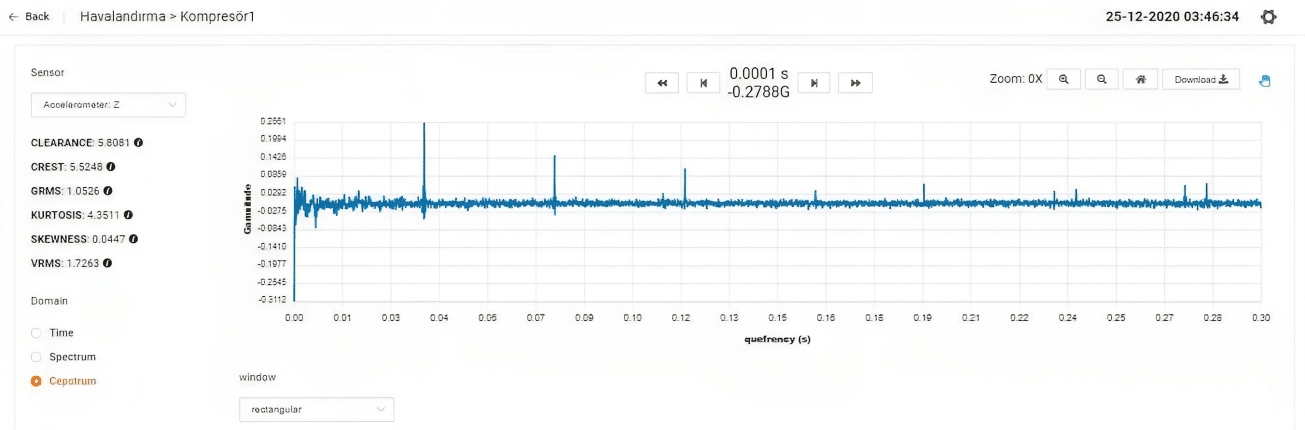
Fig. 1 Sensemore Cepstrum Dashboard
Case Study
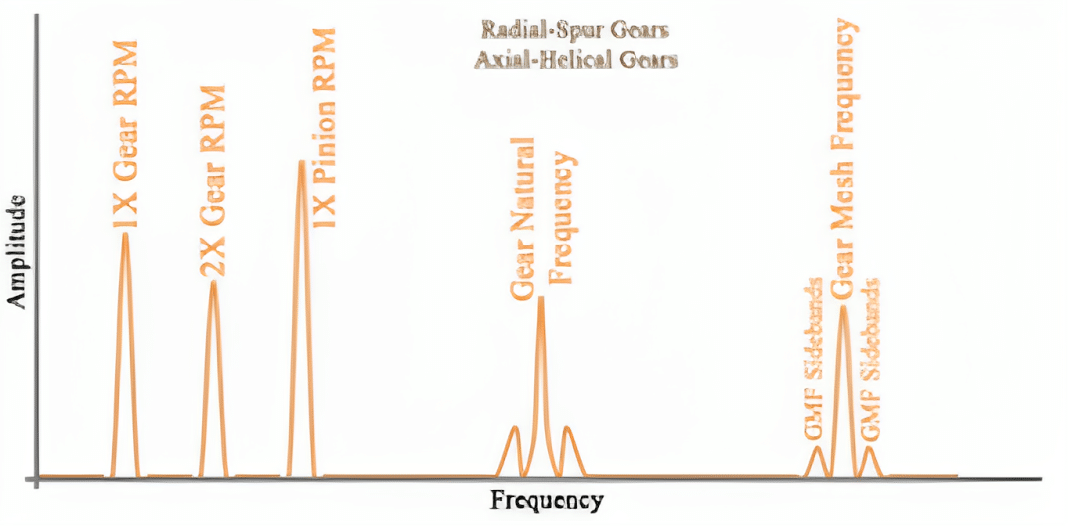
To better understand the process, let’s explain with an example. Suppose a 14-tooth pinion is attached to a motor rotating at 3000 RPM, and the pinion gear is turning the output gear, which has 42 teeth. In this case, the gear mesh frequency will be:
GMF = 3000 x 14 = 42000 RPM
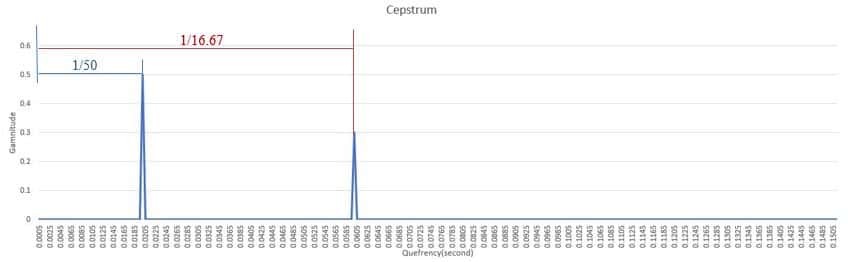
Since sidebands will be produced at gear rotation speeds, sidebands are produced at 3000 RPM and 1000 RPM.
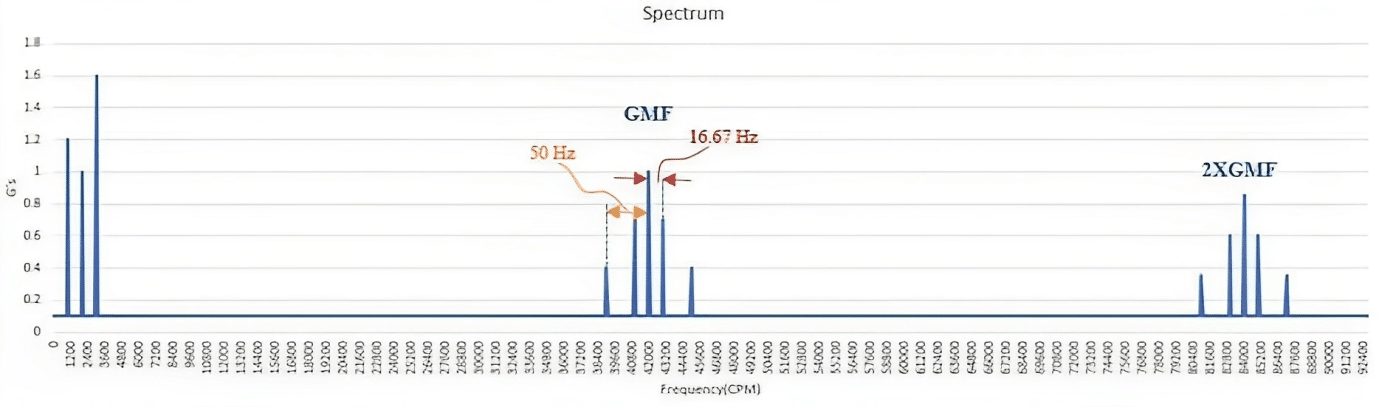
These sideband spacings that are produced in the cepstrum can be easily detected. The cepstrum has a horizontal axis with units of seconds. To determine the corresponding frequency, a 1/second calculation is made. The severity of the gear fault is directly proportional to the magnitude of the amplitudes in the cepstrum.
Conclusion
The cepstrum is affected by many factors such as noise level in the spectrum, filter bandwidth and shape, and sideband spacing. In the meantime, the cepstrum is a useful tool because changes in the spectrum are not immediately visible. It also provides a nice trend graph and alarm telemetry in condition monitoring systems, especially for gearbox conditions. Therefore, the cepstrum warns of upcoming malfunctions in advance and thus creates more time for planning maintenance outages. On the other hand, it constitutes a valuable diagnostic technique for detecting and improving modulation resources during the machine development phase.